FY2016 Annual Report

Mathematical Soft Matter Unit
Professor Eliot Fried

Abstract

Following its rapid growth during FY2015, FY2016 has been a year of consolidation and sustained productivity for the MMM unit.

Scientifically, activity within the unit focused on a broad spectrum of topics. In addition to continuing ongoing efforts to (i) use molecular dynamics simulations to determine equilibrium shapes and stored energies of Möbius bands made from graphene, (ii) explain Marangoni-driven propulsion on liquid surfaces, (iii) analyze stability and bifurcation phenomena in structures made from magnetic balls, and (iv) develop a theoretical framework for studying the mechanics of unstretchable two-dimensional media like paper, we began and made significant progress on (v) the invention and investigation of novel mechanisms with limited degrees of freedom, (vi) the growth of diamond films for applications in microfluidics, neuronal cell culture, and surface-acoustic-wave devices, (vii) the hierarchical assembly of triblock copolymer in solution, and (viii) the hydrodynamics of sedimenting cylindrical and Möbius-like rings. Additionally, we were awarded a Proof of Concept project entitled "New Contactless Magnetic Couplings for Applicationsin Microfluidics, Renewable Energy, and Nautical Propulsion".

1. Staff

As of March 31, 2017

Dr. Eliot Fried, Professor
Dr. Ryohei Seto, Group Leader
Dr. Nicolas Moreno Chaparro, Postdoctoral Scholar
Dr. Wei Chung Chen, Postdoctoral Scholar
Dr. Giulio Giusteri, Postdoctoral Scholar
Dr. Tuan Hoang, Postdoctoral Scholar
Dr. Stoffel Janssens, Postdoctoral Scholar
Dr. Abdul Majid, Postdoctoral Scholar
Dr. Johannes Schoenke, Postdoctoral Scholar
Dr. Yoichi Takato, Postdoctoral Scholar
Dr. David Vazquez Cortes, Technician
Mr. Pak Too Chan, Graduate Student
Mr. Vikash Chaurasia, Research Intern
Ms. Naoko Tokumoto, Research Unit Administrator

Alumni

Mr. Ching-Hsiu Hsu, Research Intern
Mr. Antonio Martiniello, Research Intern

2. Collaborations

2.1 Unstretchable two-dimensional materials

Type of collaboration: Joint research
Researchers:
- Professor Yi-Chao Chen, University of Houston, Houston, TX, USA
- Professor Roger Fosdick, University of Minnesota, Minneapolis, MN, USA

2.2 Mechanisms with limited degrees of freedom

Type of collaboration: Joint research
Researchers:
- Professor Shizuo Kaji, Yamaguchi University, Yamaguchi City, Yamaguchi, JAPAN

2.3 Fabrication of diamond-based surface-acoustic-wave devices

Type of collaboration: Joint research
Researchers:
- Professor Satoshi Fujii, Okinawa National College of Technology, Naha, Okinawa, JAPAN

2.4 Levitation of evaporating liquid droplets on liquid substrates

Type of collaboration: Joint research
Researchers:
- Dr. Satoshi Koizumi, National Institute for Materials Science, Tsukuba, Ibaraki , JAPAN

2.5 Upscaling of self-motile suspensions by spatial averaging

Type of collaboration: Joint research
Researchers:
- Dr. Denis F. Hinz, Kamstrup A/S, Skanderborg, Denmark
- Professor Alexander Panchenko, Washington State University, Pullman, WA, USA

2.6 Fracture networks in particulate systems Type of collaboration: Joint research

Type of collaboration: Joint research
Researchers:
- Professor John E. Dolbow, Duke University, Durham, NC, USA
- Yingjie Liu, DukeUniversity, Durham, NC, USA
- Professor Mahesh Bandi, OIST, Okinawa, Japan
- Aryesh Mukherjee, OIST, Okinawa, Japan

3. Activities and Findings

3.1 Solution of the Kirchhoff–Plateau problem

Liquid films spanning rigid frames have been of longstanding interest to physicists and mathematicians, thanks to the sheer beauty of the countless observable shapes. After the experimental investigations of Plateau (1849), anticipated by Lagrange’s (1867) theoretical treatment of the minimal surface problem, the first satisfactory proofs of the existence of a surface of least area bounded by a fixed contour were provided only in the twentieth century by Garnier (1928), Radó (1930), and Douglas (1931). This formed a basis for a wealth of mathematical investigations regarding minimal surfaces, concerning various aspects and generalizations of the classical Plateau problem. An important generalization to the situation in which the boundary of the minimal surface is not fixed but is constrained to lie on a prescribed manifoldwas initially treated by Courant (1940) and Lewy (1951), whose work spurred a number of important mathematical contributions, as reviewed by Li (2015). An existence theorem for a complementary generalization, in which part of the boundary is fixed and the remaining part is an inextensible but flexible string, was later proved by Alt (1973). In this work article, we introduce a problem which combines those generalizations. We consider situations in which the boundary of the minimal surface lies on a deformable manifold, namely the surface of an elastic loop. The filament forming the loop is assumed to be thin enough to be modeled faithfully by a Kirchhoff rod, an unshearable inextensible rod which can sustain bending of its midline and twisting of its cross sections. We establish the existence of an equilibrium shape that minimizes the total energy of the system under the requirement that the bounding loop not penetrate itself while allowing for points on its surface to come into contact. In our treatment, the bounding loop retains a finite cross-sectional thickness and volume. The liquid film is, on the other hand, represented by a set with finite two-dimensional Hausdorff measure. Moreover, the region where the liquid film touches the surface of the bounding loop is not prescribed a priori. Our mathematical results substantiate the physical relevance of the model. Indeed, no matter how strong is the competition between surface tension and the elastic response of the filament from which the bounding loop is made, the system is always able to adjust to achieve a configuration that complies with the physical constraints encountered in experiments.

**Figure 3.1:** Soap films contained within a flexible loop exert a force on the loop, causing it to change shape. For instance, the same loop can form a star shape or a swan shape depending on the surface tension of the soap film.

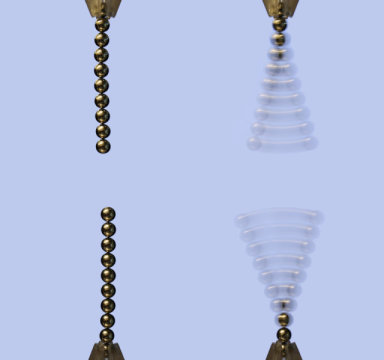
3.2 Stability of vertical magnetic chains

In modern physics it is a rare to encounter a macroscopic, globally interacting many-body system which can be manipulated directly in our hands, watching and feeling its response, while at the same time having access to an accurate yet simple theory that encompasses its behavior. In this work, we conducted a linear stability analysis for a pair of coaxial vertical chains made from permanently magnetized balls under the influence of gravity. While one chain rises from the ground, the other hangs from above, with the remaining ends separated by a gap of prescribed length. In addition to considering various boundary conditions, we account for situations in which the magnetic dipole moments in the two chains are parallel or antiparallel and we study, as a particular special case, the problem of a single chain attached to the ground. We investigate the stability of the system is examined in terms of three parameters: the number of balls in each chain, the length of the gap between the chains, and a single dimensionless parameter which embodies the competition between magnetic and gravitational forces. We also derive asymptotic scaling laws involving these parameters. Moreover, we find that it is possible to compute the Hessian matrix in exact form and thus to determine the critical parameter values at which the system loses stability and the respective eigenmodes up to machine precision. For simple a single chain attached to the ground, we obtain excellent agreement between theory and experiment.

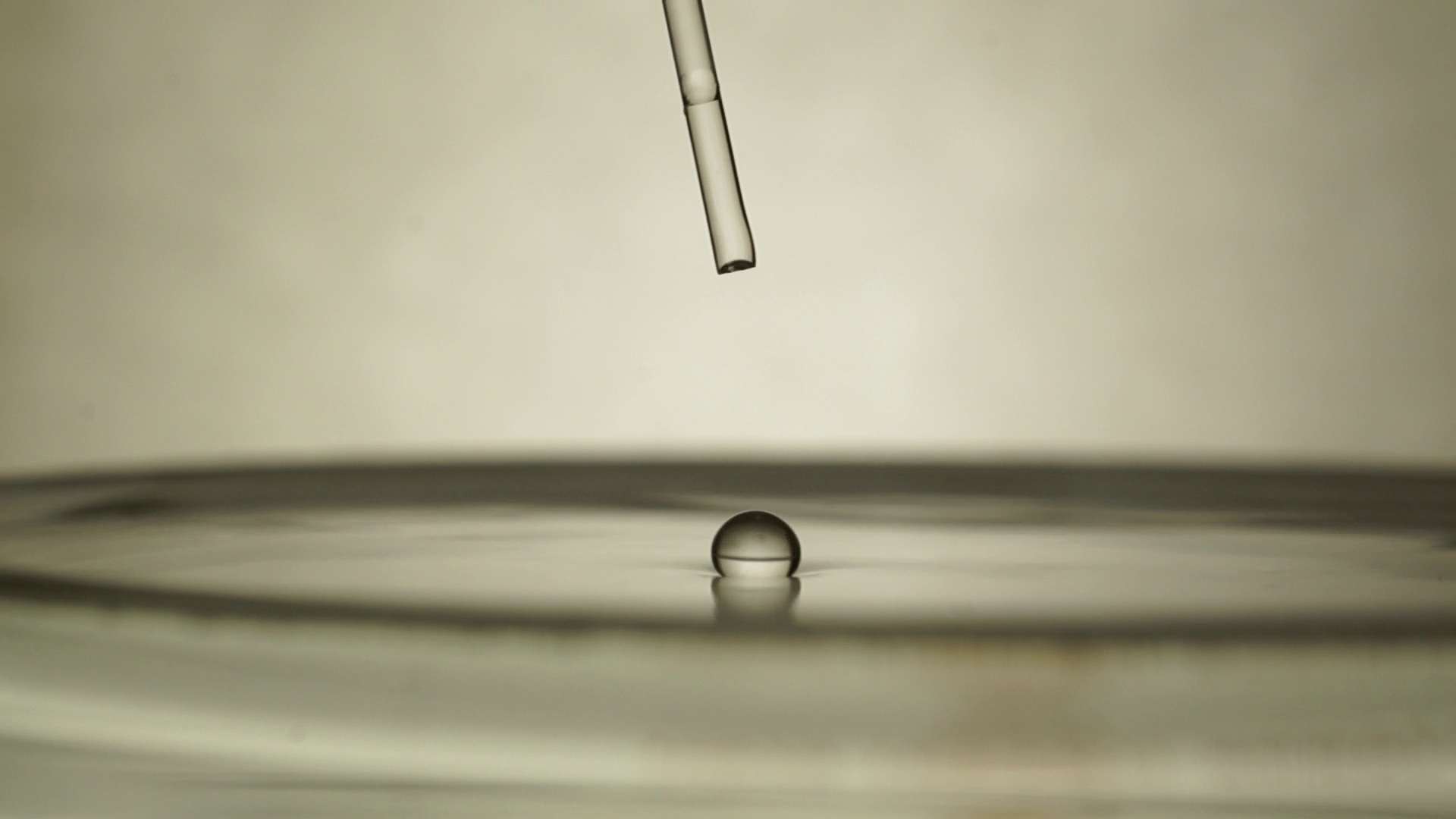
**Figure 3.2:** Stability of chains made from magnetic balls of diameter 5 mm, mass 0.5 g and magnetic flux density 1.19 T. A stack of no more than nine balls is stable (left, center) but a stack of 10 balls buckles under its own weight (right).

3.3 Behavior of self-propelled acetone droplets in a Leidenfrost state on liquid substrates

The Leidenfrost effect is a physical phenomenon where most often a droplet of water floats on a layer of steam that is formed where it meets a solid hot surface. In this work, we describe the behavior of acetone droplets in a Leidenfrost state on warm water surfaces. Acetone has a boiling point of 56ºC, well below that of water, and therefore evaporates strongly when it approaches a warm water surface, which lays at the basis of our observations. By measuring the vertical positions of hovering droplets, we deduced that the liquid surface under a droplet has a surface tension that is similar to that of acetone. This is because the acetone vapor that is produced by the droplet mixes with the water. As a consequence if this, a droplet exhibits self-propulsion. We also observed that a self-propelled droplet gradually becomes immersed beneath the undisturbed water surface. This immersion starts when a droplet has a horizontal speed of about 14 cm/s, which is accompanied by drag. This motivates us to conjecture that walking creatures such as water striders, water spiders, and rove beetles might exploit drag by immersion for locomotion. The paper derived from this work was highlighted as an Editor's Pick on the homepage of the journal, Physics of Fluids, where the paper appeared. The paper was also selected for a press release from the American Institute of Physics.

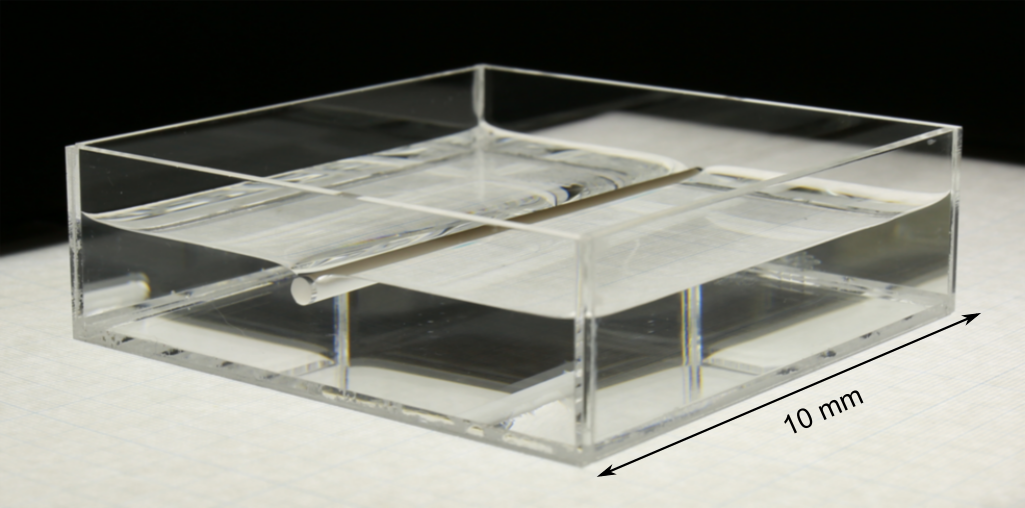
**Figure 3.3:** A 2 mm diameter acetone droplet in the Leidenfrost state on a 70ºC water bath.

3.4 Effect of surface tension imbalance on a partly submerged cylinder

The figure below shows a rectangular vessel filled with water in which a stationary cylinder is partly submerged and attached to the walls of the vessel. We applied a surfactant — an organic molecule that decreases surface tension — onto the surface of the water on one side of the cylinder, thereby allowing us to measure the profiles of the surfactant-free and the surfactant-laden portions of the water surface. We observed that the water surface curves towards the cylinder and that the curvature directly depends on the surface tension. With a surfactant on one side of the cylinder, the water surface profiles on each side of the cylinder are asymmetric due to the different curvatures. In this work, we calculated the horizontal component of the force that acts on the cylinder due to this asymmetry. Simplified models assume that the surface of the water remains completely flat on both sides of the cylinder. Despite the complexity of problem, our work reveals that the horizontal component of force is not influenced by curvature. With our findings, we can estimate that the acceleration from rest of a water walking beetle that excretes a surfactant to escape from predators is about two times the gravitational acceleration on earth. The photograph shown below was selected as the cover image of the volume of the Journal of Fluid Mechanics in which the paper based on this work appeared.

**Figure 3.4:** Photograph of a rectangular vessel filled with water in which a stationary cylinder is partly submerged and attached to the walls of the vessel.

3.5 Continuum balances from extended Hamiltonian dynamics

In this work, we applied an upscaling procedure originated by Irving and Kirkwood and refined by Noll to extended Hamiltonian systems that are employed in certain molecular dynamics simulations. This procedure makes it possible to obtain macroscopic balance laws of continuum mechanics that can be regarded as specific collective counterparts of the extended Hamiltonian dynamics of a system of material points. Our study demonstrates that the presence of auxiliary degrees of freedom, needed to control the statistical properties at the atomistic level, affects the structure of the balance equations at the continuum level. Precisely, the modifications induced at the microscopic scale influence the thermodynamic and transport properties at the macroscopic scale by way of novel source and flux terms in the continuum balances for the mass and energy densities. Our findings are particularly relevant when considering computational schemes aiming at connecting the atomistic and continuum scales. Indeed, we view it as essential to correctly subsume the statistical properties imposed on the microscopic model on the thermodynamic and transport properties of the coupled continuum. When developing multiscale numerical schemes that aim at coupling, in a physically consistent manner, statistical information retrieved from molecular dynamics with continuum modeling, this goal can be achieved by adopting continuum mechanical balances of the type we derived. We surmise that a failure to do so may lead to undesirable inconsistencies and computational artifacts.

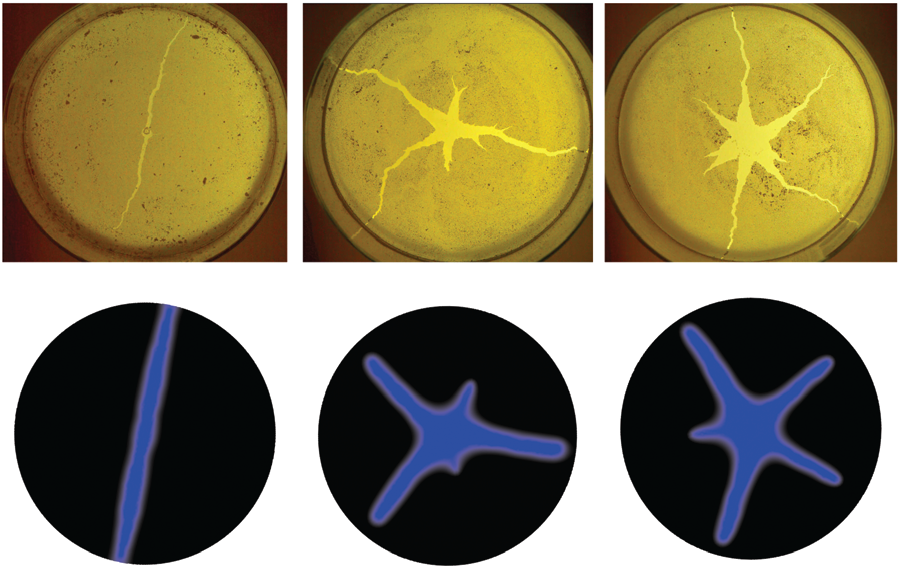
3.6 Influence of surface tension in the surfactant-driven fracture of particulate monolayers

In this work, we developed a simple continuum model that captures the main features of the surfactant-driven fracture of closely-packed particulate monolayers. The model naturally gives rise to a dimensionless parameter that can be written solely in terms of the surface tension with and without surfactant. This parameter can be used to establish a straightforward comparison with experimental conditions. New experiments were conducted to validate the model and delineate the regimes of fracture pattern as a function of the dimensionless parameter. Consistent with experimental evidence, the model and experimental incorporates a competition between the spreading force of the surfactant and the fracture toughness of the monolayer. We find that the number of fracture branches is governed by that competition.

3.7 Hierarchical assembly of triblock copolymer in solution

The hierarchical self-assembly of block copolymers in solution is a successful bottom-up methodology to design decorated (or patchy) nanoparticles with prescribed topologies and shapes. It is known that these nanoparticles can aggregate into a variety of larger microscale objects. Nevertheless, the full understanding of the critical balance between thermodynamic and kinetic factors that govern the nanoparticle-formation process and aggregation is still elusive. In this work, we use theoretical and computational models to investigate the assembly of triblock copolymers into nanoparticles. Figure 1 presents the methodology we use to create the patchy nanoparticles in multiple steps by changing the solvent affinity with the different blocks. Varying the interactions and length of the blocks we construct the phase diagram for triblock copolymers (see Figure 1.B). Our computational results are consistent with the characteristic nanoparticle shapes experimentally identified. The characteristic shapes, sizes, and distributions of the patches displayed by these subunits are crucial when it comes to controlling the growth of the microstructures. In Figure 1.C, we depict the schematic of the hierarchical process of assembly for triblock copolymers. The precise control of the aggregation at each level of process is necessary to ensure the properties of the mesoscale structures. Our results stand to accelerate the discovery and design of new materials, as well as overcome the prohibitive cost of trial-and-error methodologies.

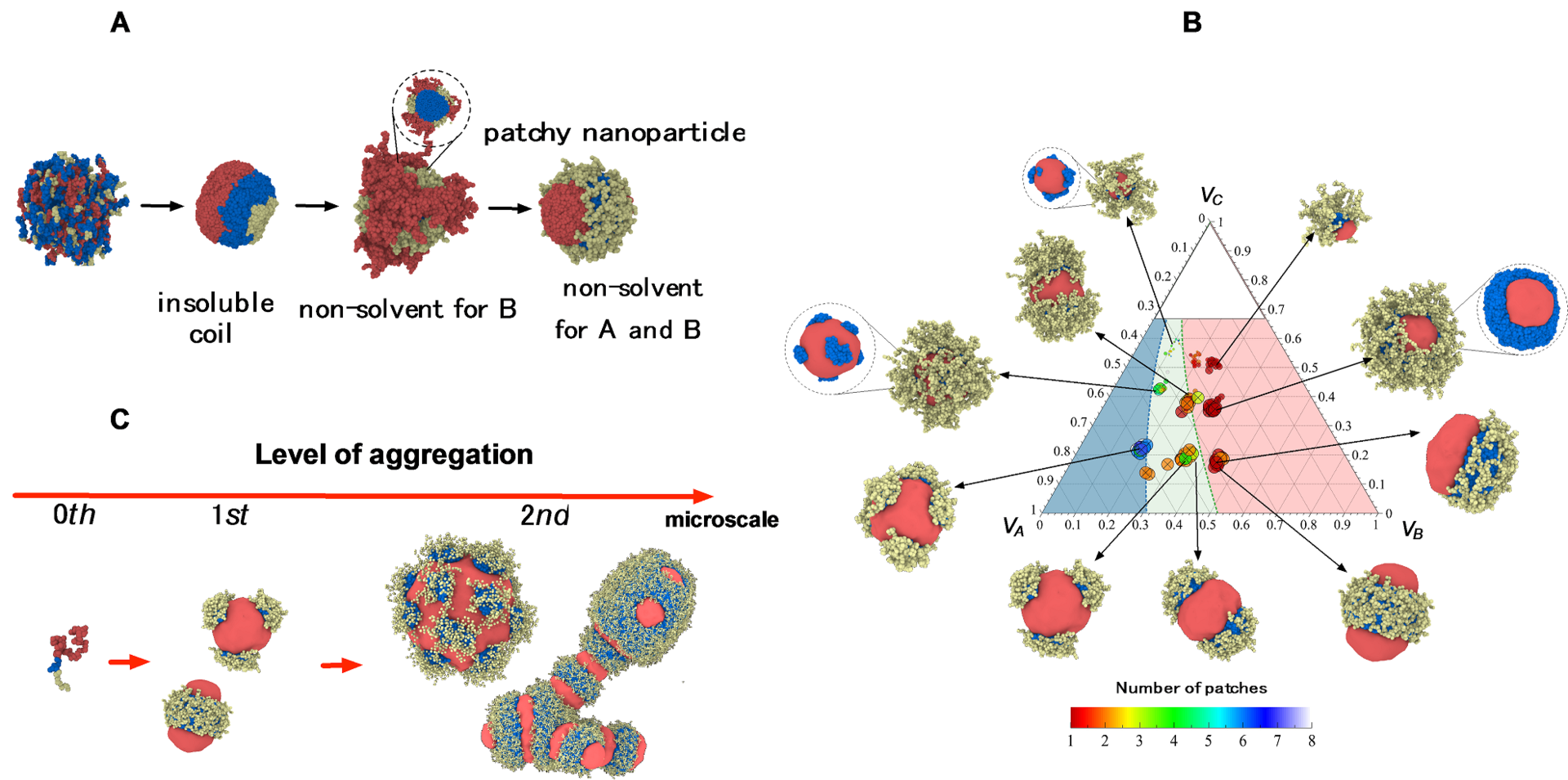
3.8 Hydrodynamics of cylindrical and Möbius-like rings

The flow behavior of colloidal dispersions is controlled by a balance between thermodynamic and hydrodynamic interactions. The thermodynamic interactions are governed by the chemical compositions of the species in the system, whereas the hydrodynamic interactions are greatly dominated by the morphology of the colloidal particles. In this project, we study experimental and computationally the hydrodynamic interactions of ring-like objects with different topologies and shapes. In particular, we compare non-orientable-like objects, or Möbius bands (rings with half a twist) with their orientable counterparts, non-twisted rings. Different aspect ratios and flow regimes are analyzed to extract the dominant modes of motion. We identify that with certain changes of shape it becomes possible to incorporate rotational motion, in a precise fashion. Furthermore, objects with chiral properties and can be used as prototypes for passive swimmers, and chiral-tracing in medical or microfluidics applications. Figure 1.A shows the sedimentation paths of Möbius bands of different aspect ratios, paths which exhibit characteristic tumbling, rotation, and rotation. Larger translation is induced at larger aspect ratios. In Figure 1.B, we depict the computational model results comparing cylindrical and Möbius-like objects. Consistent with the experimental results, twisted-rings trajectories follow helicoidal paths that increase their radius as the aspect ratio of the bands increases.

3.9 Computational study of dense suspension rheology

In this work, we developed a computational tool to be able to simulate the rheological behavior in the bulk of a dense suspension of spherical particles in a linearly viscous fluid. In particular, by implementing Lees--Edwards and Kraynik--Reinelt periodic boundary conditions, we were able to investigate the properties of the system in both simple shear and extensional flows. Dense suspensions are non-Newtonian fluids that exhibit strong shear thickening and normal stress differences. Using numerical simulations, we showed how rheological properties are determined by the microstructure that is built under flows and by the interactions between particles. In (a) of the figure, the pair distribution function highlights the presence of strong ordering below thickening for monodisperse suspensions under simple shear, and its absence above thickening. (b) No obvious ordered structure can be associated with the pair distribution function for the case of extensional flow both below and above thickening. (c,d) The polar plot of the angular distribution of contacting (and nearly contacting) particles shows that the strong enhancement of contact interactions is mainly responsible for the thickening effect. By imposing simple shear and extensional flows, we find that it is possible assess the degree of flow-type dependence in regimes below and above thickening. Even when the flow-type dependence is hindered, non-dissipative responses, such as normal stress differences, are present and characterize certain non-Newtonian features of dense suspensions. This research is the first computational study of the rheological behavior of suspensions under different imposed flows in the dense regime. Our findings form the basis for successive extensions of the analysis to flows of mixed type and help elucidating the role of the flow-induced microstructure in determining non-Newtonian effects.

Figure 3.9:
(a) The pair distribution function $g(\bm{r})$ highlights the presence of strong ordering below thickening ($\dot{\varepsilon}/\dot{\varepsilon}_0 = 0.01$) for monodisperse suspensions ($\phi = 0.54$) under simple shear, and its absence above thickening ($\dot{\varepsilon}/\dot{\varepsilon}_0 = 0.02$).
(b) No obvious ordered structure can be associated with $g(\bm{r})$ for the case of extensional flow both below and above thickening.
(c,d) The polar plot of the angular distribution $g_{\mathrm{c}}(\theta)$
of contacting (and nearly contacting) particles such that $|\bm{r}| < 2.02 a$ shows that the strong enhancement of contact interactions is mainly responsible for the thickening.

3.10 Theoretical and computational study of strongly coupled open quantum systems

Transport in quantum networks is often affected by the interplay of different environments. Typically, the effect of probability-absorbing baths is taken into account by adding non-Hermitian terms to the Hamiltonian of the system and other environments are modeled by appropriate super-operators included in the master equation. The action of both types of environment usually enters the master equation additively. The basis of the assumption is that the two baths affect the system independently and do not interfere with each other. In this effort, we investigated the limit of validity of such an assumption by means of both analytical derivations and numerical simulations. To this end, we analyzed a simple quantum network (which is a paradigmatic model for transport phenomena, as illustrated in the figure) under the influence of two different baths: a probability-absorbing environment, represented by a lead, and a dephasing environment, modeled by white-noise fluctuations of the site energies. Our results show that the additive approximation is valid if the strength of the time-dependent energy fluctuations is small compared to the bandwidth of the probability-absorbing bath. In this case, the master equation for the open quantum system can be obtained by adding the contributions generated by a non-Hermitian Hamiltonian, encoding the loss of probability, and by a Haken–Strobl super-operator, representing the dephasing bath. In the opposite regime, the breakdown of the additive assumption leads to distinctive features such as the counterintuitive suppression of the decay when the coupling to the dephasing bath is increased.

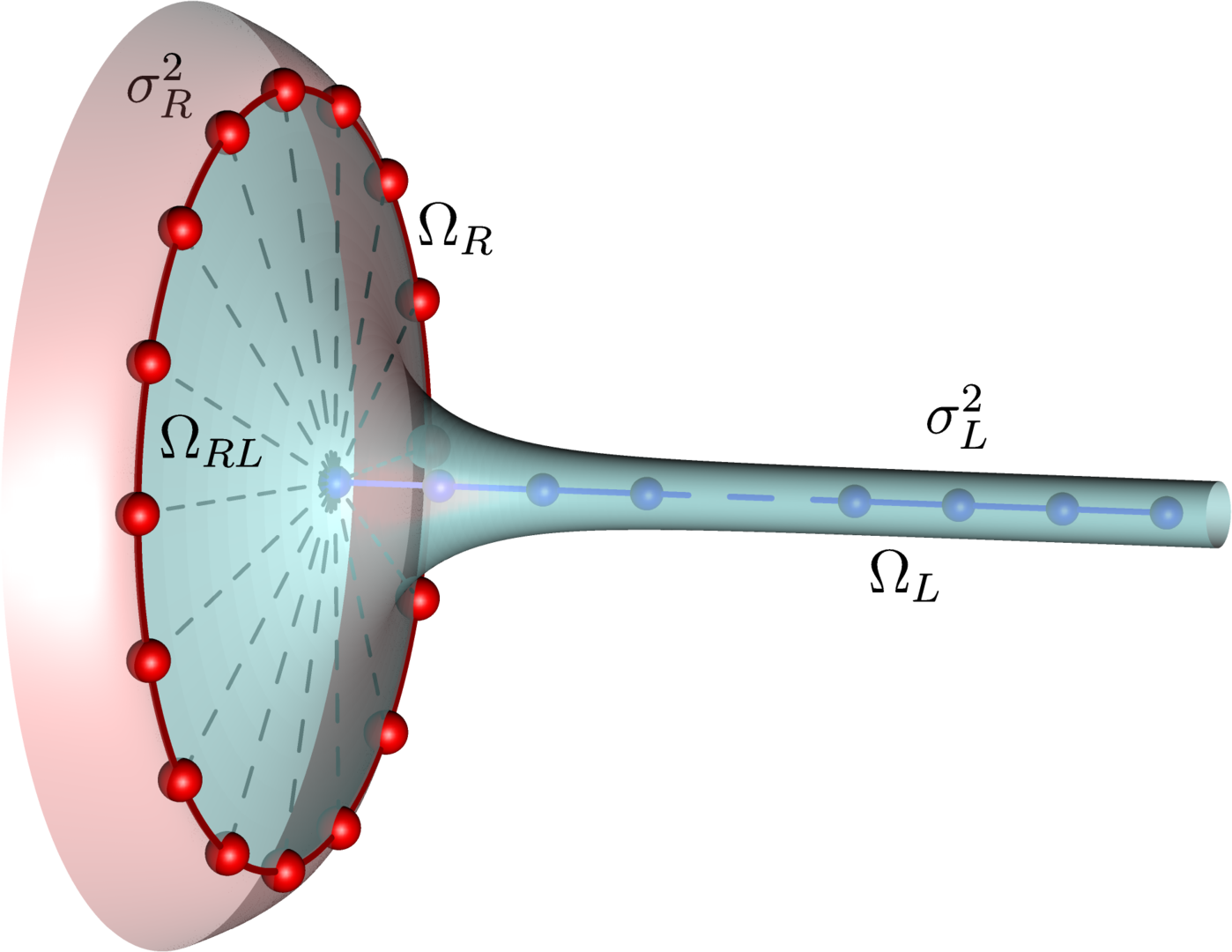
Figure 3.10: A ringlike network interacts with a dephasing bath (light red) and a probability-absorbing bath (light blue). The on-site energies of each of the $N_R$ sites of the ring, connected with nearest-neighbor coupling $\Omega_R$, undergo white-noise fluctuations with dephasing strength $\sigma_R^2$. The probability-absorbing bath is modeled by a lead of $N_L$ sites, connected with nearest-neighbor coupling $\Omega_L$. The ring sites are equally coupled to the first lead site with tunneling amplitude $\Omega_{RL}$ (dashed lines).

4. Publications

4.1 Journals

S.D. Janssens, S. Koizumi, E. Fried. Behavior of self-propelled acetone droplets in a Leidenfrost state on liquid substrates, Physics of Fluids 29 (2017), 031203. (doi:10.1063/1.4977442)
J. Schönke, E. Fried, Stability of vertical magnetic chains, Proceedings of the Royal Society of London Series A, Mathematical, Physical and Engineering Sciences 473 (2017), 20160703. (doi:10.1098/rspa/2016.0703)
G. G. Giusteri, L. Lussardi, E. Fried, Solution of the Kirchhoff–Plateau problem, Journal of Nonlinear Science 27 (2017), 1043–1063. (doi:10.1007/s00332-017-9359-4)
A. T. Pham, R. Seto, J. Schönke, D. Y. Joh, A. Chikotli, E. Fried, B. B. Yellen. Crystallization kinetics of binary colloidal monolayers, Soft Matter 12 (2016), 7735–7746. (doi:10.1039/c6sm01072e)
G. Schaller, G.G. Giusteri, G.L. Celardo, Collective couplings: Rectification and supertransmittance, Physical Review E 94 (2016) 3. (doi: 10.1103/PhysRevE.94.032135)
Y.-C. Chen, E. Fried. Möbius bands, unstretchable material sheets, and developable surfaces, Proceedings of the Royal Society of London Series A, Mathematical, Physical and Engineering Sciences 472 (2016), 20150760. (doi:10.1098/rspa.2016.0459)
N. Rotundo, T.-Y. Kim, W. Jiang, L. Heltai, E. Fried. Error estimates of a B-spline based finite-element method for wind-driven ocean circulation, Journal of Scientific Computing 69 (2016), 430–459. (doi:10.1007/s10915-016-0201-1)
G. G. Giusteri, P. Franceschini, E. Fried. Instability paths in the Kirchhoff–Plateau problem, Journal of Nonlinear Science 26 (2016), 1097–1132. (doi:10.1007/s00332-016-9299-4)

4.2 Books and other one-time publications

Nothing to report

4.3 Oral and Poster Presentations

E. Fried, Solution of the Kirchhoff-Plateau problem, PIMS (Pacific Institute for the Mathematical Sciences), The University of British Columbia, Canada, June 16 (2016).
E. Fried, Distinction between the physical constraint of unstretchability and the geometrical notion of developability, Department of Mechanical Engineering, University of British Columbia, Canada, June 20 (2016).
G.G. Giusteri, Instability paths in the Kirchhoff–Plateau problem, EPFL (École polytechnique fédérale de Lausanne), Switzerland, May 23 (2016)
R. Seto, Discontinuous Shear Thickening Fluid in a Wide-Gap Couette Cell, Kyoto University, Japan, June 29 (2016)
R. Seto, R. Mari, J. F. Morris, M.M. Denn, E. Fried, Shear thickening: SD-DEM model for dense suspensions, WCCM XII & APCOM VI, Soeul, Korea, July 27 (2016)
R. Seto, R. Mari, J. F. Morris, M.M. Denn, E. Fried, How do Discontinuous Shear Thickening Suspensions Flow in a Wide Gap Couette Cell?, XVIIth ICR Kyoto 2016, Japan, August 10 (2016)
R. Seto, A.T. Pham, B.B. Yellen, E. Fried, Grain boundary kinetics during crystallization in magnetic colloidal monolayers, New Aspects of Micro- and Macro-scopic Flows in Soft Matters, OIST, Japan, August 16 (2016)
E. Fried, Geometry and Physics of Bendable but Unstretchable Two-Dimensional Elastic Bodies, University of Calgary, Canada, August 19 (2016)
T. Hoang, E. Fried, Stability and buckling of a flat circular, intrinsically curved filament spanned by a fluid film, ICTAM 2016, Montreal, Canada, August 22 (2016)
J. Schoenke, Stability of vertical magnetic chains, 16w5021 - Mathematical Problems of Orientationally Ordered Soft Solids, Ozxaca, Mexico, September 7 (2016)
R. Seto, Quasi-static Motion and Mechanics in Colloidal Dispersions (コロイド分散系の準静的運動と力学), Kanazawa University, Ishikawa prefecure, Japan, September 11 (2016)
R. Seto, A.T. Pham, B.B. Yellen, E. Fried, Grain boundary kinetics during crystallization in magnetic colloidal monolayers, JPS 2016 Autumn meeting, Kanazawa University, Ishikawa Prefecture, Japan, September 13 (2016)
G.G. Giusteri, The shapes of a rod are traced in a Lie algebra, Geometry and Materials Sciences, OIST, October 15 (2016)
J. Schoenke, Multi trajectory expansion for the constraint motion of mechanism, Geometry and Materials Sciences, OIST, October 15 (2016)
R. Seto, Nonuniform flow of shear thickening suspensions in widegap rotary Couette geometry, CoMFoS16: Mathematical Analysis of Continuum Mechanics and Industrial Applications II, Kyusyu University, Fukuoka, Japan, October 22 (2016)
E. Fried, Geometric variational problems involving competition between line and surface energy, CoMFoS16: Mathematical Analysis of Continuum Mechanics and Industrial Applications II, Kyusyu University, Fukuoka, Japan, October 23 (2016)
E. Fried, Shape transitions in some systemes involving surface and line energy, Stanford, USA, November 11 (2016)
E. Fried, Stokesian dynamics of colloidal suspensions in extensional flow, University of California, Berkeley, USA, November 14 (2016)
E. Fried, Finite-time singularity during the collision of two magnetic balls, Santa Clara University, USA, November 15 (2016)
E. Fried, Solution of the Kirchhoff-Plateau Problem, NCTS (National Center for Theoretical Sciences), Taipei, Taiwan, December 2 (2016)
E. Fried, Stokesian dynamics of colloidal suspensions in extensional flow, National University of Singapore, Singapore, December 5 (2016)
E. Fried, Shape transitions in some systems involving surface and line energy, Department of Civil and Environmental Engineering, National University of Singapore, Singapore, December 6 (2016)
E. Fried, Shape transitions in some systems involving surface and line energy, Mechanobiology Institute, National University of Singapore, Singapore, December 7 (2016)
E. Fried, Finite-time singularity during the collision of two magnetic balls, Singapore University of Technology and Design, Singapore, December 8 (2016)
G.G. Giusteri, Paths in the special Euclidean algebra and rod shapes, NCTS (National Center for Theoretical Sciences), Taipei, Taiwan, January 13 (2017)
E. Fried, Existence of energy minimizing solutions to the Kirchhoff - Plateau problem, James Serrin: From his legacy to the new frontiers, Perugia, Italy, February 1 (2017)
E. Fried, Stokesian dynamics of colloidal suspensions in extensional flow, University of British Columbia, Canada, February 16 (2016)
V. Chaurasia, Interacting elastic curves on a rigid manifold, Mini Symposium: Viscoelasticity and Dissipative Dynamics of Rods and Membranes, OIST, Japan, March 8 (2017)
G.G Giusteri, Dimensional reduction of viscoelastic continua, Mini Symposium: Viscoelasticity and Dissipative Dynamics of Rods and Membranes, OIST, Japan, March 8 (2017)
R. Seto, Thickening in extensional flow: Toward non-Newtonian fluid model for dense suspensions, Poster Presentation, Non-Gaussian fluctuation and rheology in jammed matter, Yukawa Institute for Theoretical Physics (YITP), Japan, March 9 (2017)
R. Seto, 固体の流れ方---高濃度粒子分散系の局所レオロジーと連続体モデル, Earchquake Research Institute, The University of Tokyo, Japan, March 30 (2017)
S.D. Janssens, V. Chaurasia, E. Fried, Effect of a surface tension imbalance on a partly submerged cylinder, Poster presentation, APS March meeting, New Orleans, Louisiana, USA, March 15 (2017)
S.D. Janssens, E. Fried, Behavior of self-propelled droplets in a Leidenfrost state on liquid substrates, APS March meeting, New Orleans, Louisiana, USA, March 15 (2017)