FY2020 Annual Report
Mathematics, Mechanics, and Materials Unit
Professor Eliot Fried

Abstract
FY2020 was a productive year for the Mathematics, Mechanics, and Materials Unit. During this period, unit members published 12 peer-reviewed journal articles and a US patent. They also kept busy with an unprecedented number of diverse outreach activities in Okinawa. Unit members also devoted expertise and time to design and fabricate more than 2000 face shields for the Okinawa Provincial Government. A mobile Möbius kaleidocycle sculpture installed in the entrance hall of Laboratory 4 marks the culmination of an effort spanning more than two years and displays the connections between mathematics, engineering design, and art. During a 5-month sabbatical leave, the unit leader initiated a host of new collaborations in diverse areas of fluid and solid mechanics, several of which have already led to the submission of peer-reviewed journal articles.
1. Staff
As of March 31, 2021
- Dr. Eliot Fried, Professor
- Dr. Johannes Schönke, Staff Scientist
- Dr. Stoffel Janssens, Staff Scientist
- Dr. Vikash Chaurasia, Postdoctoral Scholar
- Dr. Adel Fernando Sarmiento Rodriguez, Postdoctoral Scholar
- Dr. Burhannudin Sutisna, Postdoctoral Scholar
- Dr. Nicholas Mario Wardhana, Postdoctoral Scholar
- Dr. Vishesh Bhat, Postdoctoral Scholar
- Dr. Smriti, Postdoctoral Scholar
- Dr. James Kwiecinski, JSPS Postdoctoral Fellow
- Dr. David Vazquez Cortes, Research Unit Technician
- Mr. Michael Grunwald, Research Unit Technician
- Mr. Dmitrii Koldaev, Graduate Student
- Mr. Alexandru Mihai, Graduate Student
- Mr. Ali Rahmani, Graduate Student
- Ms. Akyl Shakir, Graduate Student
- Mr. Sandeep Rajendra Kumar, Graduate Student
- Mr. Sutashu Tomonaga, Research Intern
- Ms. Naoko Tokumoto, Research Unit Administrator
Alumni
- Dr. Alessandro Giussani, Postdoctoral Scholar
- Mr. Martín Forsberg Conde, Visiting Research Student
- Ms. Aliyah Osman, Research Intern
2. Collaborations
2.1 Unstretchable two-dimensional materials
- Type of collaboration: Joint research
- Researchers:
- Professor Yi-Chao Chen, University of Houston, Houston, TX, USA
- Professor Roger Fosdick, University of Minnesota, Minneapolis, MN, USA
- Professor Brian Seguin, Loyola University Chicago, IL, USA
2.2 Fabrication of diamond-based surface-acoustic-wave devices
- Type of collaboration: Joint research
- Researchers:
- Professor Satoshi Fujii, Okinawa National College of Technology, Naha, Okinawa, JAPAN
2.3 Modeling of size-dependent crystal plasticty in TRIP steel
- Type of collaboration: Joint research
- Researchers:
- Professor Takeshi Iwamoto, Academy of Science and Technology, Hiroshima University, JAPAN
2.4 Flows of thin films on curved substrates
- Type of collaboration: Joint research
- Researchers:
- Professor Daisuke Takagi, University of Hawaii at Mānoa
2.5 Fluid flows in gel networks
- Type of collaboration: Joint research
- Researchers:
- Professor Fernando Duda, Federal University of Rio de Janeiro
- Professor Angela Souza, Federal University Fluminense
2.6 Effects of incompatibility in fluid flows
- Type of collaboration: Joint research
- Researchers:
- Professor Roger Fosdick, University of Minnesota
2.7 Microscopic models for the rheological properties of novel suspensions
- Type of collaboration: Joint research
- Researchers:
- Professor A. Jeffrey Giacomin, Queen's University
- Ms. Mona Kanso, Queen's University
2.8 Randersian geometry and thermodynamics
- Type of collaboration: Joint research
- Researchers:
- Professor Manolis Vevakis, Duke University
- Dr. Martin Lesueur, Duke University
- Mr. Alexandre Guével
3. Activities and Findings
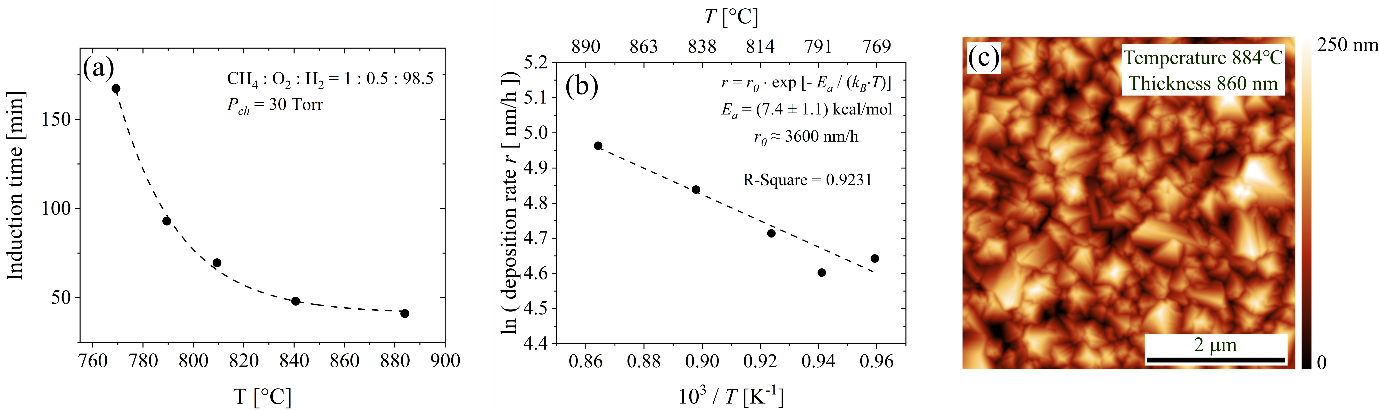
3.1 The interaction between denotation nanodiamond seeds and oxygen-enriched plasmas in the microwave plasma enhanced chemical vapor deposition of nanocrystalline diamond films on silicon substrates
High quality nanocrystalline diamond (NCD) films can be grown by the microwave plasma enhanced chemical vapor deposition technique, employing diluted mixtures of a hydrocarbon in hydrogen as the gas source. The addition of an oxygen admixture to the gas feed is known to abate the incorporation of impurities but poses technological challenges to the onset of film growth.
We used a gas mixture of 1 vol% methane and 0.5-1.5 vol% oxygen in hydrogen with the goal of depositing NCD films on (001)-oriented silicon substrates seeded with detonation nanodiamonds of 5·10-2 density. We studied how the oxygen-enriched plasma affects the detonation nanodiamonds and we identified the space of deposition parameters that allow for NCD film growth. For a reactor pressure of 30 Torr and an oxygen admixture of 0.5 vol%, for instance, the time necessary to induce film growth decreased exponentially with the substrate temperature as shown in Fig. 1a, and the deposition rate exhibited an Arrhenius dependence on the substrate temperature with an apparent activation energy of 7.4~kcal/mol, as portrayed in Fig. 1b. The atomic force microscope image of the surface of a ~ 860 nm-thick NCD film deposited at 30 Torr and 884°C from a gas mixture with [O2] = 0.5 vol% is presented in Fig. 1(c). The rms roughness is ~ 25 nm.
For chamber pressures higher than 30 Torr and for high concentrations of oxygen, either the induction time becomes impractically long for any application or the seeds are completely etched by the oxygenated plasma and no film growth occurs at all. To circumvent this issue and expand the growth window, we developed a two-step process, in which the oxygen additive is supplied after an NCD precursor layer has been deposited from an oxygen-less gas feed.
3.2 Nanoscale control of nanodiamond arrays in polymeric matrices
In this study, we investigated an unprecedented strategy for avoiding nanodiamond (ND) self-agglomeration and for directing the nanoscale assembly of NDs by tailoring molecular interactions between block copolymers (BCPs) and functionalized NDs. The project was motivated by the challenge in guiding NDs into arrays, which is highly relevant for the development of advanced hybrid materials and quantum devices.
We have presented a simple and scalable approach to form hybrid micelles by establishing H-bond interactions between an amphiphilic triblock terpolymer and carboxyl-functionalized NDs. Co-assembly between NDs and the terpolymer culminated in nanocomposite films with a remarkably uniform distribution of NDs at a high ND loading exceeding 50 wt%, which has never been previously reported, in addition to film transparency and UV-protective capability.
We further showed the formation of a network-like superstructure with well-aligned NDs via a stepwise co-assembly method. A complex interplay between the terpolymer self-assembly, terpolymer–ND interactions, and ND colloidal behavior took a significant role in the structure formation. Our findings on BCP-guided assembly of NDs may lay an important foundation for further discoveries of hierarchically ordered nanocomposites, with the potential to benefit a wide range of disciplines from biology to computer science.
3.3 Complex viscosity of a suspension of Möbius macromolecules
In this paper, we use general rigid bead–rod theory calculate the complex viscosity and the corresponding first normal stress coefficient of suspension of Möbius macromolecules undergoing small-amplitude oscillatory shear flow. We discover binary behaviors for both the real and imaginary parts of the complex viscosity. By binary, we mean that the result depends only on the twist parity of the Möbius macromolecule. In contrast, we discover that the behaviors for all three parts of the first normal stress coefficients in small-amplitude oscillatory shear flow are not binary.
3.4 Entropic factors and structural motifs of triblock-terpolymer-based patchy nanoparticles
In this work, we focus on exploring how entropic factors and geometrical constraints that influence the formation of different structural motifs during the synthesis of triblock- terpolymer-based patchy nanoparticles. Additionally, we demonstrate how intrinsic geometrical constraints lead naturally to parameters that can be used to achieve target morphologies. Our description in terms of these parameters shows that the attainable motifs correspond to ground states that reflect a balance between interfacial and conformation entropic contributions. Our theoretical predictions are confirmed by matching matching existing experimental results for previously discovered morphologies and by our own experiments for newly discovered morphologies. We provide a library of soft patchy nanoparticles comprised the family of structures that can be assembled independent of detailed polymer chemistry. Elements of this library, which are freely available on Bitbucket as data in LAMMPS format (see: https://bitbucket.org/nmorenoc/patchy-nanoparticles/src/master/), can be used for designing hierarchically assembled mesostructures.

3.5 The two basic notions of curvature for a surface in three space dimensions
The two basic notions of curvature for a surface in three space dimensions are the mean and Gaussian curvatures. Minimal surfaces, which have zero mean curvature, have been studied extensively for both their distinct mathematical properties and their remarkable prominence in applications. Developable surfaces. which have zero Gaussian curvature — are also of particular significant since they can be smoothly flattened onto a plane without stretching or contraction. The work required to deform sheets of materials as diverse as paper, leather, metal, and glass into developable shapes is much less than that needed to attain other shapes. Developable surfaces have thus been of longstanding relevance, including the design of ship hulls and automobile parts, lightweight structures like facades and pavillions, apparel, and footwear. Due to the widespread utility of developable surfaces, methods for generating and processing them are now standard features of most computer-aided design platforms. Apart from devising algorithms for animating the bending of paper and origami and the draping and folding of cloth, computer scientists have discovered a host of novel applications for developable surfaces, including the segmentation and parametrization of meshes and the mapping of textures. Most recently, mechanisms that can be placed on developable surfaces and, thus, allow for compact storage have been realized. There are two familiar constructions of a developable surface from a space curve. We demonstrate that there is a continuous family of developable surfaces that can be generated from a curve, one surface for each function that is defined on the curve and has magnitude less than or equal to the curvature of the curve. We also that the geodesic curvature of the curve relative to each such constructed surface is precisely equal to the choice of the mentioned function.
3.6 Closed unstretchable knotless ribbons and the Wunderlich functional
In 1962, Wunderlich published the article "On a developable Möbius band", in which he attempted to determine the equilibrium shape of a free standing Möbius band. In line with Sadowsky's pioneering works on Möbius bands of infinitesimal width, Wunderlich used an energy minimization principle, which asserts that the equilibrium shape of the Möbius band has the lowest bending energy among all possible shapes of the band. By using the developability of the band, Wunderlich reduced the bending energy from a surface integral to a line integral without assuming that the width is small. Although Wunderlich did not completely succeed in determining the equilibrium shape of the Möbius band, his dimensionally reduced energy integral is arguably one of the most important developments in the field. In this work, we provide a rigorous justification of the validity of the Wunderlich integral and fully formulate the energy minimization problem associated with finding the equilibrium shapes of closed bands, including both orientable and non-orientable bands with arbitrary number of twists. This includes characterizing the function space of the energy functional, dealing with the isometry and local injectivity constraints, and deriving the Euler–Lagrange equations. Special attention is given to the connecting edge conditions, regularity properties of the deformed bands, determination of the parameter space needed to ensure that the deformation is surjective, reduction of isometry constraints, and matching conditions and jump conditions associated with the Euler–Lagrange equations.

3.7 Kaleidocycle Sculpture "Floating Ring" at Lab4 entrance
On 31 March 2021 the installation of the first Möbius Kaleidocycle sculpture, a self-moving meter-scale object, was finished in the main entrance hall of Lab 4. In a more than two year effort, the sculpture was conceived and designed in the MMMU and realized in close collaboration with Archimedes Exhibitions Berlin.
Installation of the Möbius Kaleidocycle sculpture in March 2021.
4. Publications
4.1 Journals
- M. Kanso, V. Chaurasia, E. Fried, A.J. Giacomin. Peplomer bulb shape and Coronavirus rotational diffusivity, Physics of Fluids 33 (2021), 033115-1–7. (doi:10.1063/5.0048626)
- J. Schönke, M. Grunwald, E. Fried. Construction of unknotted and knotted symmetric developable bands, Symmetry 13 (2021), 431-1–18. (doi:10.3390/sym13030431)
- B. Sutisna, S.D. Janssens, A. Giussani, D. Vázquez-Cortés, E. Fried. Block copolymer-nanodiamond coassembly in solution: Towards multifunctional hybrid materials, Nanoscale 13 (2021), 1639–1651. (doi:10.1039/d0nr07441a)
- B. Seguin, Y.-C. Chen, E. Fried. Bridging the gap between rectifying developables and tangent developables: a family of developable surfaces associated with a space curve, Proceedings of the Royal Society of London Series A, Mathematical, Physical and Engineering Sciences, 407 (2020), 20200617-1–14. (doi:10.1098/rspa.2020.0617)
- N. Moreno, B. Sutisna, E. Fried. Entropic factors and structural motifs of triblock-terpolymer-based patchy nanoparticles, Nanoscale 12 (2020), 22059–22069. (doi:10.1039/D0NR06192A)
- B. Seguin, Y.-C. Chen, E. Fried. Closed unstretchable ribbons and the Wunderlich functional, Journal of Nonlinear Science 30 (2020), 2577–2611. (doi:10.1007/s00332-020-09630-z)
- J.A. Kwiecinski, A. Goriely, S.J. Chapman, Interactions of anisotropic inclusions on a fluid membrane, SIAM Journal on Applied Mathematics, 80(6) (2020), 2448-2471. (doi: 10.1137/20M1332694)
- L. Espath, V.M. Calo, E. Fried. Generalized Swift–Hohenberg and phase-field-crystal equations based on a second-gradient phase-field theory, Meccanica 55 (2020), 1853–1868. (doi:10.1007/s11012-020-01228-9)
- J.H. Piette, N. Moreno, E. Fried, A.J. Giacomin. The complex viscosity of Möbius macromolecules, Physics of Fluids 32 (2020), 093107-1–13. (doi:10.1063/5.0022546)
- A. Majid, Simulation of a soap film spanning a flexible loop, International Journal of Non-Linear Mechanics, 124 (2020), 103497-1–8. (doi:10.1016/j.ijnonlinmec.2020.103497)
- J.A. Kwiecinski, Relaxation of viscoelastic tumblers with application to 1I/2017 U1 ('Oumuamua) and 4179 Toutatis, Monthly Notices of the Royal Astronomical Society, 497 (1) (2020), 19-36. (doi: 10.1093/mnras/staa1933)
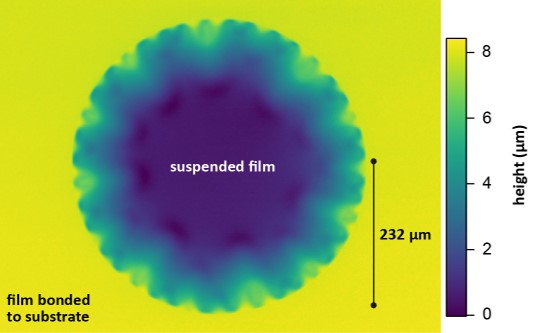
- S.D. Janssens, B. Sutisna, A. Giussani, J.A. Kwiecinski, D. Vázquez-Cortés, E. Fried. Boundary curvature effect on the wrinkling of thin suspended films, Applied Physics Letters 116 (2020), 193702-1–5. (doi:10.1063/5.0006164)
5. Intellectual Property Rights and Other Specific Achievements
5.1 Patent Application
Title: Method of fabricating a through glass via on a suspended nanocrystalline diamond
Inventors: Dr. Stoffel Janssens, Dr. David Vazquez-Cortes, Dr. Alessandro Giussani, Prof. Eliot Fried
Publication date: November 5, 2020
Link: https://patentscope2.wipo.int/search/en/detail.jsf?docId=WO2020222297
5.2 JSPS KAKENHI: Grant-in-Aid for Early Career Scientists
Postdoctoral Fellow: Dr. Burhannudin Sutisna
Period: From April 2020
5.3 JSPS Postdoctoral Fellowship for Research in Japan
Postdoctoral Fellow: Dr. James Kwiecinski
Period: From September 2019 to August 2021
6. Meetings and Events
6.1 Meetings
6.1.1 Seminars
Dr. Martin Skrodzki (iTHEMS program, RIKEN)
- Date: November 10, 2020
- Venue: L4E01, Lab 4, OIST Campus, and Zoom
- Seminar: Piecewise Linear Simplifications of Geometries: Variational Shape Approximation of Point Set Surfaces
6.1.2 Long Term Visiting Researcher
- Professor Yi-chao Chen, University of Houston
- Professor Daisuke Takagi, University of Hawaii, Manoa
6.2 Events
6.2.1 GMP2020
This international conference was initially scheduled in the middle of May, 2020. However, due to Covid19 pandemic, it was postponed to September, and eventually be held as a briefing online session.
- Date: September 23, 2020
- Venue: Online
7. Other
7.1 A publication "Boundary curvature effect on the wrinkling of thin suspended films" has been shared on International and Domestic Media
Related links:
- OIST News Center: Scientists iron out the physics of wrinkling ( English | Japanese )
- 7th Space
- Bioengineer
- Phys.org
- Fabcross.jp
- ScienceDaily
- Azo Materials
- SciTechDaily
7.2 3D Printed Face Shields: Mr. Michael Grunwald
Face shields for the prefectural government, a collaboration with Shen unit and multiple volunteers from OIST. Part of OIST’s Covid response.

to block coronavirus droplets and aerosols.
7.3 Science Outreach Activity for Highschool in Australia: Dr. James Kwiecinski
Dr. Kwiecinski wrote and provided about mathematically modeling the spread of Covid19, which is entitled "When Science and Mathematics Collide: Modeling the Covid-19 Pandemic."
7.4 Talk at Kyuyo High School: Dr. Johannes Schönke
- Title: "Möbius Tables and Kaleidocycles: New Ideas for Furniture and Mechanical Linkages"
- Date: October 30, 2020
- Venue: Kyuyo High School, Okinawa-city, Okinawa

7.5 Workshop in Aguni island: Dr. Johannes Schönke
- Title: "Mechanism"
- Date: December 14, 2020
- Venue: Aguni island, Okinawa





