[Seminar] The SOKOBAN random walk by Ofek Lauber Bonomo
Date
Location
Description
Abstract:
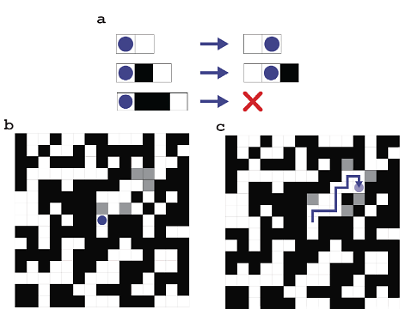
Random motion in disordered media is sensitive to the presence of obstacles, preventing atoms, molecules, and other particles from moving freely in space. When obstacles are static, a sharp transition between confined motion and free diffusion occurs at a critical obstacle density: the percolation threshold. In this talk, I introduce the Sokoban random walk, which is a new type of random walk, designed to test if this conventional wisdom continues to hold in the presence of simple tracer-media interactions. Akin to the protagonist of an eponymous video game [1], the Sokoban has some ability to push away obstacles that block its path. I show that while one expects this will allow the Sokoban to venture further away, it is surprisingly not always the case. Indeed, as it moves – pushing obstacles around – the Sokoban dynamically confines itself to a finite region whose mean size is uniquely determined by the initial obstacle density [2] . This finding breaks from the ruling “ant in a labyrinth” paradigm, vividly illustrating that even weak and localized tracer-media interactions cannot be neglected when coming to understand transport phenomena.
[1] Sokoban: https://www.mathsisfun.com/games/sokoban.html
[2] Bonomo, O.L. and Reuveni, S., 2022. Loss of Percolation Transition in the Presence of Simple Tracer-Media Interactions. arXiv preprint arXiv:2210.04343.
Subscribe to the OIST Calendar: Right-click to download, then open in your calendar application.



